A. INTRODUCTION:
RADAR is an acronym for Radio Detection And Ranging. In all of the radar units which have been (and are being) utilized by the NWS, a great deal more than simply "detection" and "ranging" have taken place. Over the years, technological advances in materials, circuit design, high-speed devices, processing capabilities, and observations have combined to allow radar systems to be significantly improved.
A good case in point is the WSR-57, long the stalwart of the nationwide network of NWS weather radar systems. A number of modifications to the WSR-57 have both extended its useful operational life and allowed it to perform in ways which the original designers could never have conceived. As an example, the '57 has been interfaced with digital processing technology and modern communications systems which allow the radar data to be displayed and transmitted far beyond its three original console CRTs and camera scope.
In the early 1960s, H. W. Hiser wrote: "In the future, it is likely that small, solid-state, 'off-the-shelf-hardware' digital computers will be used for on-line, real-time analysis of data at the radar for local use and for temporary storage of the digital data on magnetic tape prior to transmission elsewhere."
It seems that we have arrived at a point in time (and technology) in which we might say that we have a radar system such as the one Hiser described nearly thirty years ago. That system is the WSR-88D, a radar and communications system that was literally born out of the minds of Hiser and others.
In order to ensure a solid foundation from which to study the
WSR-88D system, a measure of knowledge of the fundamental principles of
radar is a necessity. The discussions in this set of prerequisite
radar lessons are intended to provide a review of those fundamentals.
Topics included will be reflected waves, pulsed waves, radar beamwidth,
propagation, pulse length, pulse repetition frequency, polarization, target
resolution, beam paths, pulse volume, and echoing volume.
B. REFERENCES:
Radar Principles, (NWSTC MRRAD410, 1988)
Fundamentals Of Weather Radar Systems, (NWSTC MRRAD420, 1990)
Radar Meteorology, (H.W. Hiser, Third Edition, 1970)
C. DISCUSSION:
An electromagnetic wave may be represented in space as shown in the figure below. Also shown is the radio transmitter, receiver, and an obstruction to the wave. (Later, we'll refer to this obstruction as a "target").
If emitted toward the obstruction, the waves strike it, and
a certain portion of the energy (much less than the total energy impinging
on the obstruction) is reflected back toward the transmitter. In
actuality, what occurs is that the waves are "scattered" many directions
from the surfaces of the obstruction which was struck by the wave.
If the obstruction in the drawing happened to be a cloud of water droplets, the transmitted (incident) wave would be "scattered" in nearly every direction by each of the droplets. It may be said that each droplet of water would "re-radiate" the energy which it received from the transmitted wave. Further, each droplet acts much like a small dipole antenna. If the droplets vary in size, so will their antenna electrical characteristics vary accordingly. Some droplets will radiate more energy than will others. The maximum amount of this re-radiation will, of course, be determined by the size of the droplet and by the wavelength of the incident radiation. Due to the spherical shape of the droplets, re-radiation takes place in all directions (scattering). The aircraft in the drawing will produce scattered re-radiation as well. Its shape and size will, of course, determine the pattern of scattering.
The total amount of reflected energy (in any direction) is dependent upon several factors, some of which will be discussed in this unit. Suffice to say here, if we transmit ordinary electromagnetic waves which strike some obstruction, a very small amount of this energy will be reflected (re-radiated) back toward the point of transmission.
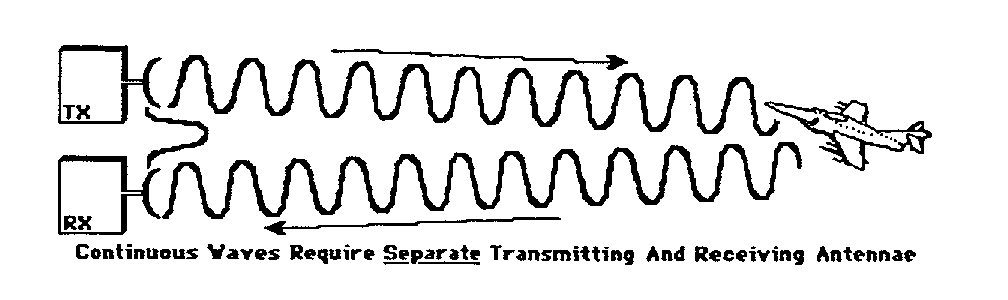
In a continuous wave system, such as the one discussed above,
it would seem that any reflected waves which return toward the transmitter
will be cancelled or obscured by interference from outgoing waves.
If this occurs, there can be no method by which detection of the
reflected energy could be accomplished. In order to permit
the use of a single antenna, and to measure the distance from the
antenna to the reflecting surface(s), the "pulsed" wave radar system
was developed, and is to be the exclusive subject of our discussion.
The drawing below depicts the "pulsed" waves of a radar system.
Note that there is a single antenna. This figure, while greatly exaggerated
in the time domain, shows both the "interval" between the wave pulses and
the duration of the pulse itself.
The pulse duration is called the "pulse length", and is measured in micro-seconds (one micro-second is one-millionth of a second). The pulse length is usually called the PULSE WIDTH in radar systems.
The pulse interval, or the time from the beginning of one pulse to the beginning of the next, is determined by the number of pulses which are transmitted in a given period of time. In radar, we measure all time in seconds (or fractions of seconds). As a result, the equation for measuring the pulse interval time is....
One Second
Pulse Repetition Interval =
__________________
# Of Pulses Per Second
If we were to transmit 1000 pulses in a second, the interval
time from the beginning of one pulse to the beginning of the next would
be...
1
Pulse Repetition Interval =
___________ = 0.001 Seconds
(PRI)
1000
The time interval is known as "PRI", and also frequently called
"PRT". The number of pulses transmitted in one second is called the
"frequency", and is most often referred to as the "PRF" (pulse repetition
frequency).
The "duty ratio" (often called the Duty Cycle) is the ratio
of the pulse width (PW) to the pulse repetition frequency (PRF), and is
given by...
Duty Cycle = PW * PRF
... where the PW is in seconds, and the PRF is
in pulses per second.
The duty cycle expresses the ratio of transmitter "ON" time
to the total available time (PRI). If we use our PRF example
above (1000 pulses per second), and each pulse emitted was one micro-second
(0.000001 seconds), the duty cycle value would be...
Duty Cycle = 0.000001 * 1000
= 0.001
This means our transmitter is actually "ON" for one one-thousandth of the total time measured. One method to use in understanding the meaning of "duty cycle" is indeed an interesting one. Take a look at your watch, and, as the second hand passes the exact beginning of any HOUR (1 o'clock, 2 o'clock, etc), scream at the top of your lungs for precisely 3.6 seconds. Then, wait in silence until the exact beginning of the NEXT HOUR, and then repeat the 3.6 second scream. The ratio of your screaming and silence will be exactly the same ratio as our 1000 Hz PRF and 1 micro-second pulse width above.
What does the duty ratio really mean in terms of a radar system? Since each of the transmitted pulses contains a definite amount of energy (and the same amount of energy is contained in each pulse), the duty cycle is a value which allows us to calculate the energy (power) of a single pulse as if the power had been evenly spread throughout the total time from the beginning of one pulse to the beginning of the next.
Again, consider your hourly "scream". If your 3.6 second yell energy was to be "averaged" over the entire hour, how loud would the noise be? Of course, the little "hum" that results might be quite difficult to hear.
Likewise, if our 1 micro-second pulse contained a million watts
of power, what average power would result if it was to be averaged over
the period of time allowed by our 1000 Hz PRF?
The answer is found by multiplying the power in the pulse by
the duty cycle (Peak Power * Duty Cycle) ...
1,000,000 Watts * 0.001 = 1,000 Watts
(Peak Power) * (DC) = (Average Power)
It should be obvious that the duty cycle is the "ratio" between
the PEAK energy in the radar pulse and the AVERAGE energy expended
over a specified period of time. Since the devices we
use to measure radar waves are "averaging" devices, we need to be
able to express PEAK power in terms of the AVERAGE power which results
from pulsing energy on and off. This applies to circuits both in the radar
transmitter and in the radar receiver.
The "beam" of energy is accomplished by using an antenna which
focuses the radar energy onto a parabolic reflector. A common analogy
to this is found in an ordinary flashlight. The polished reflector
found in a flashlight has the effect of directing the light waves in a
concentrated "beam". The beam of light may be directed in any desired
direction, so that we might "illuminate" objects with the bright
(powerful) energy radiated from the flashlight. If you direct the
flashlight on a wall, you will see a bright "spot" at the center of the
"beam" of light. It is apparent that most of the light energy is
focused in that small area.
And so it is with a radar "beam". The parabolic antenna reflector has the same effect on the radio-frequency electro-magnetic waves emitted by the transmitter. The intent is to focus the energy into a narrow beam so that greater "illumination" of objects of interest may be accomplished. This greater "illumination" results in more energy being reflected back to the point of origin. In the case of the flashlight, you see much more light reflected from the "spot", and in the case of the radar, a great deal more energy is reflected from the strongest concentration of waves (the center of the beam).
In theory, the paraboloid shape of the antenna reflector should result in a "pencil" beam. However, diffraction at the edges of the antenna dish (related to the wavelength) cause the beam to become slightly "conical", and results in a slight spreading of the beam as the energy travels away from the antenna. This spreading causes a linear variation in the physical width of the beam as the transmitted pulse progagates away.
The width of the radar energy "beam" is a critical factor in many of the calculations which are needed to determine the amount of energy that is detected in the pulses reflected from weather "targets". Because the parabolic antenna is unable to focus all of the wave energy at the exact center of the beam, some of the transmitted power of the wave is spread away from the center axis of the beam. At some distance (and angle) from the beam axis, this power can be found to be half of the power measured at the axis. This distance, or angle, is said to be a ½-power point. There are an infinite number of ½-power points located around the center of the beam. Theoretically, each of these points should contain a power level which is half of that at the center. Since ½-power may also be represented by the term -3dB, these points are often referred to as the -3dB points. The width of the beam, relative to two of these points located 180 apart, is called the ½ power (or the -3dB) beamwidth. The beamwidth is expressed as the angle theta ( ), as determined by...
71.6 Wavelength
Beamwidth (0 ) =
___________________
Antenna Diameter (d)
...where 0 is in degrees ( o ),
and wavelength and antenna diameter are in the same units (feet,
inches, meters, centimeters, etc.). If we use centimeters as our
reference wavelength in the formula, then we also must use centimeters
as the antenna diameter (d) in the formula.
As an example, the WSR-57 antenna is 12 feet (3.657 meters)
in diameter, and the wavelength is (for 2885 MHz) 10.3986 centimeters.
Calculating the beamwidth from the formula would yield...
71.6 * 10.3986
0 =
______________ = 2.036o
365.7
Repeating the calculation for the much larger WSR-88D radar
antenna diameter (@ 28 feet) and wavelength (still "S" band) results in
a very narrow ½-power beamwidth ( 0 ) of about
0.95o .
By comparison, the WSR-74C radar system (5625 MHZ and an 8 foot antenna) has a beamwidth ( ) of about 1.6o .
Yet another point regarding the concept of beamwidth must be
considered. Since the beamwidth is simply an angle ( 0
), and the beam spreads as a function of range, the physical size of the
wavefront becomes a factor when the measurement of "target" echoes must
be accomplished. As an example, the WSR-57 beam (2.0o
) spreads to the dimensions indicated in the table below.
Range (nmi)
2o Beam Diameter
______________
_________________
25
5,307 feet
50
10,613 feet
75
15,920 feet
100
21,227 feet
125
26,534 feet
150
31,840 feet
200
42,454 feet
250
53,067 feet
It should be noticed that the spreading doubles as the range
doubles. This linear relationship is true for all radar beamwidths.
Finally, because of the diffraction of the beam, only about 80% of the transmitted energy is contained in the -3dB area which we have called the beamwidth. The same action which causes the widening of the beam also causes some of the energy (about 20%) to be emitted (in lesser concentrations) at even wider angles from the antenna. These areas of energy radiation are called sidelobes. Since the beam is three-dimensional, so also are the sidelobes, as depicted in the drawing below.
Recall our "flashlight" analogy. If you pointed a flashlight toward a wall, you can see the central bright spot caused by the main beam, as expected. However, you should also see a ragged, relatively dim "ring" of light around the central bright spot. This is a sidelobe. All weather radar antennas have several sidelobes, separated by specific angles relative to the center of the main beam. The power in these lobes is considerably less than the power focused into the main beam (primary lobe), but still is sufficient to result in unwanted radar echoes from targets, especially those that are close to the radar antenna.
The drawing above shows one of the bursts of electromagnetic
waves which could be emitted by the radar transmitter. The energy
is in the form of high-frequency oscillations, the exact number of which
depend on the transmitter frequency and the pulse width (PW).
In the WSR-57 radar, using a pulse width of 4 µSeconds, the energy burst contains about 11,540 oscillations of radio-frequency energy. If we display the burst on an oscilloscope, we can only view the pulse envelope which contains the high-frequency oscillations. In our NWS radars, we can view the envelope of the radio-frequency burst by connecting a crystal detector and oscilloscope to one of the waveguide ports in the radar transmitter. We can only calculate the number of oscillations in the pulse period.
The action of the pulsed radar energy may be simply depicted
in the diagram below. In this case, our target is meteorological
in nature (a thunderstorm).
For now, we'll not discuss the details of the many variations
possible in the nature of radar "targets". Those subjects (regarding
weather radar) will be included in subsequent information sheets.
Synchronizing signals in the radar specify the precise time when the radar transmitter circuits must generate a burst of electro-magnetic energy. At the same instant, display circuits are also synchronized.
The full-power energy leaves the radar antenna, and travels (contained by the beam) toward the precipitation target. At the target, the power of the pulse has been reduced substantially. Some of the power which strikes the water droplets in the thunderstorm are re-radiated in the direction of the antenna. Again, during the return trip, the power in the pulse diminishes. The antenna collects the "echoed" energy, which is a tiny fraction of the strength of the original transmitted pulse.
In the radar receiver, the received "echo" is amplified, mixed
with a local oscillator signal, amplified more, and then converted to a
"video" voltage for display on the radar scope(s). The position of
the video voltage in the domain of measured time following the transmitter
pulse determines the distance of the target which reflected the energy.
In radar, this time is called range.
c = 300,000,000 meters per second (300,000 Km. per Sec.)
161,800 nautical
miles per second
186,420 statute
miles per second
984,300,000 feet
per second
In our discussions, velocity conversions must be done in both meters and nautical miles, since the WSR-88D system utilizes both units in measuring and in displaying weather echoes. Older NWS radar systems (WSR-57) measured in nautical miles, while the WSR-74 series systems are based on meter and kilometer distances.
The velocity of wave propagation is critical to the operation of any radar system, since measurements of elapsed time between transmitter pulses and received "echo" signals provide the only method for determining the distance between the radar and the target(s). The table below indicates the distances traveled by a radar wave in various units of time.
In the accurate measurement of time intervals in radar, we are concerned more with the time of total travel of the pulsed wave. This is the time in the right- hand column in the table on page 11, which represents the time from the instant the wave leaves the transmitting antenna until the reflected wave returns to the same antenna. To state this in another way, the reflection interval (the time in the right column) is exactly twice the time it takes the wave to reach the target. If our radar system is configured to measure (display) the radar information in nautical mile increments, we would refer to the reflection interval time 12.36 µSeconds in the right column as "one radar nautical mile". On the other hand, if the radar is set up for kilometer display increments, we would use the 6.67 µSecond value (again from the reflected interval column), and this time would be called "one radar kilometer".
At this point, one of the fundamental radar design considerations must be presented, expressed in mathematical terms. This concept is known as the the Radar Range Formula. The mathematical expression is...
ct
where... c = the speed of light
R = _____
t = the PRI (pulse interval)
2
R = range from the transmitter
As an example, consider the WSR-88D PRI (pulse repetition interval) of 3066.66 µSeconds. The Range expression would be as follows...
300,000,000 * .00306666
R = _______________________
= 460,000 meters
2
Take note (from the table on page 11) that a reflection interval time of 3,066.66 µSeconds equates to a range (distance to the target) of 460,000 meters. This is also 460 Kilometers, which is, by no coincidence, also the maximum range of the WSR-88D.
Yet another term which is often utilized in this regard is
unambiguous range. Simply stated, the unambiguous range is the greatest
distance that a radar pulse can travel and return to the radar antenna
BEFORE the next pulse is transmitted. We'll find that the WSR-88D
must be able to correct ambuguities (doubtful or uncertain information)
in range during its pulse-to-pulse task of gathering and processing meteorological
information. We'll discover that some special methods (unique to
the '88D) are utilized to resolve range ambiguiutes.
Here, as in the drawing on page 4, the "firing frequency" (PRF), the length of the "bullet" (pulse width), and the interval BETWEEN "shots" (PRI) can be readily perceived. Further, all of the energy (power) is contained in the BULLET, the amount of power delivered to the target depends upon the LENGTH of the bullet as well as on the NUMBER OF HITS on the target in a given period of time (PRF). The PRI (bullet interval) is the time measured from the beginning of one bullet to the beginning of the next.
If the shooter loads up with BIGGER (and longer) bullets, the energy reaching his target will increase proportionally if he fires at the same frequency. With regard to the radar, if the pulse width (PW) is increased (with no change in the PRF), the meteorological target will likewise receive more energy over a given period of time. This is precisely what occurs in the WSR-88D. Two pulse widths are available for transmission. These widths are 1.57 µS and 4.5 µS.
In addition, unlike the standard machine gun, the '88D can
also vary the PRF. As indicated in the attachment "PRIs" (p.26),
the PRF frequencies currently
available for the WSR-88D range from 321 Hz to 1,282 Hz.
Variation of the PRF and PW in the 88D transmitter provides superb flexibility
in maintaining control of the power which is ultimately delivered from
the antenna. This is very important in measurement of storm intensities,
and will also be vital in the 88D's ability to extract additional data
from the meteorological target(s).
The NWS WSR-57 radar uses horizontal linear polarization, A drawing of this type of wave orientation is shown below...
Notice that the polarization of the magnetic "M" field reverses
with each ½-cycle, but remains oriented vertically with respect
to the earth's surface. Because raindrops tend to become oblate (flatten
out) as they fall, weather radar systems have traditionally employed horizontal
linear polarization. This method allows improved signal returns from
weather targets.
An alternative to vertical or horizontal linear polarization
was tried in the early WSR-88D systems. This technique is called
circular polarization. In this kind of electro-magnetic emission,
the "E" field is no longer confined to a single plane, but consists of
equal-amplitude horizontally and vertically polarized components which
are phase-shifted by 90o . Refer to the diagram below...
It can be readily seen that the vectors of both the "E" and "M" fields are rotating in a clockwise direction (if viewed from behind the antenna). This rotation is called right-hand circular polarization. In the drawing, only of the wavelength ( ) is shown. Notice that the fields have rotated by 45o . After ¼ , the rotation will be 90o , and after one full , the field vectors will have completed a full 360o of rotation. So, for every cycle of the transmitted wave, the "E" and "M" fields are rotated a full 360o . The observer (standing behind the antenna) would "see" the rotation vector in this drawing rotating in a circular clockwise motion. This is the reason for utilizing the terminology "circular polarization".
The direction, either clockwise or counter-clockwise, can be
controlled by the design of the antenna feed assembly. More often
that not, CW rotation is referred to as right-hand polarization, and CCW
rotation is called left-hand polarization. Early-model WSR-88D systems
used a device called an orthogonal mode transducer (OMT) mounted in the
antenna. The OMT provided right-hand polarization. This circular
polarization scheme did not provide the desired result, and all full-production
'88D systems are fitted with antenna systems that utilize horizontal LINEAR
polarization.
If right-hand circular polarization is transmitted, the waves which are reflected back from precipitation targets are analogous to a "mirror-image". That is, the energy returns to the antenna as left-hand polarization. Since the radar uses the same antenna for both transmission and reception, the antenna is much less responsive to the opposite sense of rotation. As a result, direct reflections from spherical targets (such as round raindrops) are not readily passed through the polarizer to the receiver. However, a complex target such as an aircraft will return some energy with the correct polarization. Energy from an aircraft may be returned on one "bounce", (as from a flat plane or spherical surface), or may make two or more "bounces" between various portions of the target before returning to the radar antenna. Signals which make single reflections (or any odd number) will be generally rejected by the circularly polarized antenna.
On the other hand, signals which "bounce" twice (or any even number of times) will be fairly easily accepted. Circular polarization, therefore, has traditionally been used as a solution to the problem of rejecting echoes from symmetrical targets. Precipitation targets are generally spheroid (therefore symmetrical) in their shape, and have traditionally been rejected with circular polarization.
The ability to reject rain echoes depends on the degree of polarization circularity that can be generated by a practical antenna and on the shape of the pre- cipitation particles. In practice, it is relatively easy to acheive a high ( ~ 20dB) integrated cancellation ratio (ICR) at a single frequency, but rather difficult to do so over a range of frequencies. ICR is a "figure of merit" for a circularly polarized antenna that takes into account the polarization of the entire radar beam rather than the polarization on just the axis or peak of the beam. In effect, it is the weighted average of the cancellation ratios at each point on the beam. One factor which tends to reduce or limit the effectiveness of circular polarization is ground reflected energy, which actually changes the polarization.
The radar cross-section of an aircraft target is, in general,
less with circular polarization than with linear polarization.
It should be pointed out that the difference in echo return with circular
and linear polarizations depends heavily on the aspect (viewing angle)
of the target. Since it has been shown that circularly-polarized
aircraft echoes are somewhere between 3dB and 6dB less than with linear
polarization, air traffic control (ATC) radars utilize antenna designs
which are switchable between the two polarization techniques. If
the ATC controller wishes to view precipitation on his scope, he can switch
the radar to a linear polarization mode, somewhat at the expense of reducing
(if only temporarily) his ability to detect aircraft.
In the early '88D design, the WSR-88D radar system used the
OMT and separate waveguide feeds for transmit and receive modes, allowing
the traditional theory (as detailed in the discussion on pages 15
and 16) of circularly polarized detection to be reversed. In
these '88Ds, the mirror-image left-hand polarized echoes were passed easily
into the receiver section, while all other polarizations (including right-hand
waves) were severely attenuated. The intent was to allow the WSR-88D polarization
device to easily limit echoes from aircraft and from other non-meteorological
targets.
Notice that (with the 1 µS pulse) any target that is less than 150 meters away from the antenna could not be detected by the radar. This is due to the fact that the leading edge of the reflected wave would return to the antenna BEFORE the trailing edge is emitted. The pulse width (H) determines the minimum range at which targets can be detected. This minimum range is approximately ½ the length of the wave burst. In the case of the 4.5µS pulse, the minimum range would be 675 meters (2,215 feet). This is also equal to approximately 0.36 nautical mile. With a 1.57 µS pulse (as in the WSR-88D short-pulse mode) the minimum range would be about 235 meters. In actual practice, the minimum range of a radar is somewhat larger than the values given above because of the slight amount of delay encountered in enabling the receiver after the transmitted pulse has cleared the antenna.
In older model radars, this delay is due to the recovery time
of the T/R tube (duplexer). In the WSR-88D, the computer controls
both the firing of the transmitter (each and every pulse) and the protection
of the receiver during transmitter bursts. After sensing that the
high-power energy has diminished in the waveguide, the computer then allows
the receiver to be activated.
In the same direction of thought, consider that two (2) targets
are very close to each other and along nearly the same azimuth from the
radar. Assume further that these targets are well beyond the minimum
range of the radar, as described on page 17.
The pulse width utilized here is 1µS. Refer to the drawing below...
If the distance between the two targets is less than ½ of the pulse width (in our case, less than 150 meters), the reflected waves from both targets will be combined into one (1) composite wave. Only a relatively large target will be seen on the radar indicator. If, on the other hand, the two targets have a separation which is greater than ½ the pulse width, the received energy will return in two (2) bursts, and two separate targets will be detected on the radar indicator. It should be apparent that the pulse width has a decided effect on the target resolution in the domain of range. It follows that, logically, the shorter the pulse width, the finer the resolution of targets.
Longer pulse widths do, however, posess a certain notable advantage,
especially in meteorological applications. The 4.5µS long pulse
width will contain about 4½ times as much energy as the 1µS
pulse. This increase in energy (power) permits detection of targets
at greater ranges, and will result in the detection of weaker targets at
short range than will the 1µS pulse. Also, the longer pulse
will compensate for some attenuation of short pulse waves which prevents
full development of targets with considerable depth in range. These effects
are readily observable on current NWS radar systems which have dual pulse
width capability (WSR-57 and WSR-74S). Although the target definition
suffers somewhat in the long pulse mode, the advantages often outweigh
the disadvantages.
Early in this discussion, it was stated that electro-magnetic waves (like light waves) could be formed into "beams". The ordinary flashlight was used as an example of beamed energy. Other examples could be car headlights,searchlights, etc. Through the use of suitable antenna reflectors (paraboloids), we found that it is possible to concentrate most of the transmitter's energy into a single beam. Further, by rotating the reflector in the horizontal (azimuth) as well as in the vertical (elevation) planes, it is possible to control the direction of the beam. The direction of either beam axis (horizontal or vertical) may be displayed on the appropriate radar scope an any given instant, allowing the targets illuminated by the beam to be displayed at BOTH the correct time (range) and bearing (direction). Once again, however, the question of differentiation (resolution) of the target(s) must be addressed. Recall that, as the beamed energy travels away from the antenna, the width of the beam expands. If the radar antenna is rotating in azimuth (horizontally), a single target will appear to be stretched (subtended) in width. This is due to the fact that the energy is reflected as soon as the leading edge of the beam strikes the target, and energy continues to be reflected until the trailing edge of the beam has passed the target. The subtension of any target will be a function of the width of the beam.
As an example, refer to the WSR-57 beam diameter table on page
8. With a 2 beamwidth, the PHYSICAL WIDTH of the beam is 21,227
feet at 100 nautical miles in range. This width is nearly four (4)
miles. The reflected energy which would return from a "point" target
(an airplane, etc.) would result in a display that makes the target appear
to be nearly four (4) miles in width.
If the target was a rain shower, it also would be stretched
by the beamwidth. Since a rain shower is not a point target, the error
in apparent width would not be as dramatic. However, the beamwidth
effect would add four (4) miles to the actual width of the shower.
The same stretching occurs in the vertical (elevation) axis. Recall that the beam is symmetrical in three dimensions. When WSR-57 radar operators scan vertically through a thunderstorm to determine the height of the precipitation "tops", they must add a correction to compensate for the difference between the actual height and the apparent height which is caused by the beamwidth. (Note that the correction applied must be adjusted for the range of the target scanned.)
Now, consider the same antenna directed at two (2) aircraft
which are located close together (within one beamwidth). It is easily
seen that the energy that is reflected from each target will merge into
a composite wave that will appear on the radar scope as one (1) target.
In order for the radar to detect the presence of two (2) targets, the planes
would have to be separated by a distance which is greater than the width
of the beam at a given range. Once again, this example assumes a
"point" target. It should be understood, however, that the
same effect takes place with any targets which are within a beamwidth of
each other, and at the same range from the radar. The obvious conclusion
in this regard is that a narrow beamwidth will serve to enhance the
detection resolution of a given radar. The WSR-88D radar antenna
has a 0.95 degree beamwidth, and therefore provides a significant
improvement over older systems with wider beams. Recall that
the beam width doubles as a function of range. In the WSR-88,
the stretching effect will be half that of the WSR-57.
In the case of the WSR-57 antenna (@ 2o beamwidth),
the gain factor is about 6460 : 1. This means that any given target
which falls into the beam of the radar will receive 6,460 times as much
power than would be received if the radar was using an isotropic (omni-directional)
radiator. This gain factor is a ratio, and may be expressed in decibels
as 38.1 dB gain. The WSR-88D radar (0.95o beamwidth)
concentrates even more of the transmitter power into the
beam than does the WSR-57. The gain of the '88D antenna is about 45.5 dB. This is a ratio of 35,480 : 1, more than five times the efficiency of the WSR-57.
The antenna gain value must be considered for BOTH the transmitted wave and the received energy. In other words, relative to the isotropic antenna, the WSR-88D antenna has the effect of amplifying the transmitter power by 45 dB, as well as amplifying the reflected energy striking the antenna by 45 dB. Generally, narrow beams provide more range capability. However, if a radar scans through space with a very narrow beam, there is an increased chance that some targets might be missed altogether. This situation depends on the target, the range, the radar PRF, and the speed of antenna rotation. In the WSR-88D, the antenna movement is completely controlled by the volume coverage patterns (VCPs) mentioned on p. 26. These patterns (which are under computer control) ensure that the antenna scans the specified azimuth and elevation sequences so that the atmosphere within the range of the radar is observed and sampled in a way which minimizes the possibility of "missing" significant target returns.
It is quite apparent that the parabolic reflector in any radar
plays an important role in the ability of the radar to detect its intended
targets.
In the atmosphere, however, variations of moisture and temperature with height result in changes in the velocity of propagation of the waves. When the speed of a wave changes, the wave is "bent", and the direction of the wave changes accordingly. These direction changes are related to the "index of refraction", which is a measure of the speed of light in a vacuum divided by the speed of propagation of a wave in the atmosphere. As implied, the index of refraction is related to atmospheric parameters. However, the functional relationship itself depends upon the wavelength of the energy being propagated.
Generally, at microwave frequencies, the "refractivity"
is expressed as...
N = (n-1) * E+6
...and the following equation is a valid approximation in the atmosphere...
Since p and p decrease rapidly with height while T decreases
slowly, N will decrease with altitude. The result is that the
velocity of wave propagation increases with altitude, and the wave is bent
somewhat back toward the earth. The path curvature (C) may be calculated
using the equation C = - the rate of change of n with respect to height.
The result is that, in a "normal" atmosphere, the radar "line-of-sight"
(beam path) is an arc which has a radius of approximately 1.34 times the
radius of the earth. See drawing below...
When there are significant deviations from the "standard" atmosphere
(extreme temperature and moisture inversions), the radar beam may be bent
more sharply toward the earth or may travel within a layer (duct) due to
reflection at upper and lower boundaries. When this occurs, ground
targets may be observed on the radar display at longer than normal (sometimes
fantastic) ranges. This phenomenon is known as "anomalous propagation",
and can present the radar operator with a very difficult scope interpretation
situation.
The pulse is of a specific physical length in space, and is
contained within the -3dB points of the beam (in both horizontal and vertical
cross-sections). The shape of the pulse volume is that of a frustum of
a cone. The pulse volume will increase in size with range, due to the spreading
beamwidth. As a result of the spreading, the power density
in any part of the volume decreases as the range from the radar increases.
The energy (WSR-88D) is present during the time of either a 1.57 µSecond
pulse or a 4.5 µSecond pulse. Therefore, at the 1.57 µSecond
setting, the pulse occupies 471 meters (1,545 feet) of range along the
beam. The pulse is 0.3 miles long.
In the WSR-57 and WSR-74 radar systems, the received energy
is "sampled" by the digital video processor (DVIP) at a rate of once every
1.67 µSeconds.
This sampling interval begins at the instant the electro-magnetic
pulse leaves the radar antenna, and continues through the entire
radar range. The timing of the samples means that the practical
"echoing volume" is an element of the atmosphere which represents ¼
kilometer of range and, of course, one (1) beamwidth in in diameter.
In both of these older radars, four (4) ¼ kilometer samples are
first summed, and then averaged into a value which represents a full kilometer
of radar range. The resultant display resolution is then one
(1) kilometer in range and one (1) beamwidth in azimuth.
These displays are synchronized by the same base timing signals which control the firing of the radar transmitter. At the same instant the electro-magnetic wave leaves the transmitter, circuits in the radar display unit are energized.
The "A" scan display takes the same form as the familiar oscilloscope
display. The range between the radar and the target is displayed on the
horizontal (X) axis, and the intensity of the target is depicted on the
vertical (Y) axis. The radar location is usually located at the left
side of the display, and the maximum range is represented on the right
edge.
The "P" scan, commonly referred to as the "PPI" (plan position indicator) is probably the most familiar and universally utilized of all the radar displays. The radar location is positioned in the center of the display tube, and the maximum range is represented by the edge of a circular path whose points are all equally distant from the center of the display. The PPI "sweep" rotates about the center (origin) of the CRT in coincidence with the physical position of the transmitting antenna. The PPI display shows radar targets in both range (distance from the center of the tube) and direction (angular position from the center of the tube). The display utilizes "polar coordinate" positioning (0o to 360o of azimuth), relative to the radar location. The "E" scan, also called "RHI" (range height indicator), displays the radar targets in both range from the radar and height above the earth. Like the PPI, the RHI "sweep" rotates vertically in coincidence with the movement of the radar antenna angle. In this case, the sweep angle represents the angle of the antenna between the horizontal (0o ) and vertical (90o ) positions.
The WSR-88D system does not use any of these traditional radar displays. Instead, a "B" scan (a television type display) is used. The "B" scan monitors are similar to the PPI scope, but are much more flexible in their ability to display various degrees of data formats.
An example of the "television" type display (B scan) is shown
below...
The WSR-88D radar system uses special scan "strategies" in order to gather both reflectivity and doppler information. These scan strategies are referred to as "Volume Coverage Patterns" (VCPs). Two of these VCPs are currently designated for a mode of operation called "Clear Air Mode", and two other VCPs are used in a "Precipitation Mode" The "Precip" (also "A") mode VCPs are called VCP #11 and VCP #21. They facilitate the sampling of fourteen (14) and nine (9) unique elevation angles respectively. VCP 11 employs 16 "cuts" (only 14 angles since the two lowest angles are repeated) in five minutes, and VCP 21 performs 11 "cuts" in six minutes. VCP 11 is shown in tabular form below. Notice the SIXTEEN antenna rotations ("cuts"). Also, take note that the PRF rate and antenna slew rate are both modified at different elevations.
EL angle
Antenna
Slew
Surv.
PRF
Pulses
for each
Speed
Time
Dopp.
Rate
per
Rotation
(RPM)
(Sec)
Batch
(pps)
Deg. AZ
Attachment... "PRIs"